DIFFERENTIATION OF INVERSE TRIGONOMETRIC FUNCTIONS

Find the first derivative of f(x) = arctan x + x 2 Solution to Example 2: Let g(x) = arctan x and h(x) = x 2, function f may be considered as the sum of functions g and h: f(x) = g(x) + h(x). Hence we use the sum rule, f '(x) = g '(x) + h '(x), to differentiate function f as follows f '(x) = 1 / (1 + x 2 ) + 2x = (2x 3 + 2x + 1) / (1 + x 2 ). The inverse hyperbolic tangent tanh^(-1)z (Zwillinger 1995, p. 481; Beyer 1987, p. 181), sometimes called the area hyperbolic tangent (Harris and Stocker 1998, p. 267), is the multivalued function that is the inverse function of the hyperbolic tangent. SOLVED The partial derivatives of arctan(y/x) let w = arctan(y/x) the partial derivatives are: dw/dx and dw/dy i know that the derivative or arctan(x) is 1/(1+x^2). Calculate the derivative of the function (y = arccos xarctan x) at (x = 0.) Example 9 Using the chain rule, derive the formula for the derivative of the inverse sine function.
None of the six basic trigonometry functions is a one-to-one function. However, in the following list, each trigonometry function is listed with an appropriately restricted domain, which makes it one-to-one.- for
- for
- for
- for , except
- for , except x = 0
- for
- for
- for
- for
- arc for , except
- arc for , except y = 0
- arc for
Derivative Of Arccos
- arc
- arc
- arc
- PROBLEM 1 : Differentiate .
Click HERE to see a detailed solution to problem 1.
- PROBLEM 2 : Differentiate .
Click HERE to see a detailed solution to problem 2.
- PROBLEM 3 : Differentiate arc arc .
Click HERE to see a detailed solution to problem 3.
- PROBLEM 4 : Let arc . Solve f'(x) = 0 for x .
Click HERE to see a detailed solution to problem 4.
- PROBLEM 5 : Let . Show that f'(x) = 0 . Conclude that.
Click HERE to see a detailed solution to problem 5.
- PROBLEM 6 : Evaluate .
Click HERE to see a detailed solution to problem 6.
Some of the following problems require use of the chain rule.
- PROBLEM 7 : Differentiate .
Click HERE to see a detailed solution to problem 7.
- PROBLEM 8 : Differentiate .
Click HERE to see a detailed solution to problem 8.
- PROBLEM 9 : Differentiate .
Click HERE to see a detailed solution to problem 9.
- PROBLEM 10 : Determine the slope of the line tangent to the graph of at x = e .
Click HERE to see a detailed solution to problem 10.
- PROBLEM 11 : Differentiate arc . What conclusion can be drawn from your answer about function y ? What conclusion can be drawn about functions arc and ?
Click HERE to see a detailed solution to problem 11.
- PROBLEM 12 : Differentiate .
Click HERE to see a detailed solution to problem 12.
- PROBLEM 13 : Find an equation of the line tangent to the graph of at x=2 .
Click HERE to see a detailed solution to problem 13.
- PROBLEM 14 : Evaluate .
Click HERE to see a detailed solution to problem 14.
- PROBLEM 15 : A movie screen on the front wall in your classroom is 16 feet high and positioned 9 feet above your eye-level. How far away from the front of the room should you sit in order to have the ``best' view ? (HINT: Find the largest possible angle in the given diagram below.)
Click HERE to see a detailed solution to problem 15.
Derivative Of Arctan
Click HERE to return to the original list of various types of calculus problems.
Your comments and suggestions are welcome. Please e-mail any correspondence to Duane Kouba by clicking on the following address :
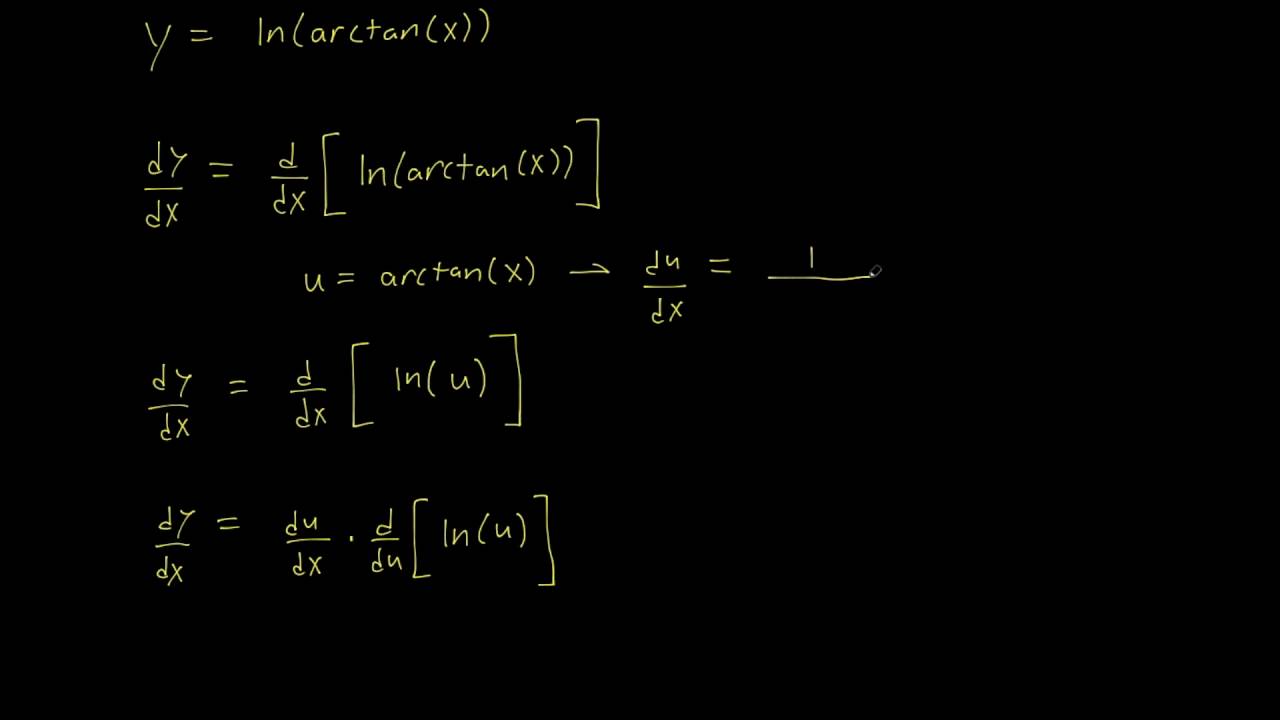
Derivative Calculator
Duane KoubaTue Sep 16 11:30:08 PDT 1997